.gif)
MENU
Methods and Extent of Present Research
![]() Wolf-Moose Coaction
Wolf-Moose Coaction
|
Fauna of the National Parks — No. 7
The Wolves of Isle Royale |

|
DYNAMICS OF WOLF-MOOSE COACTION
SINCE the approximate size and reproductive rate of the moose herd, and rate of kill by the wolves are known, deductions can be made about the long-range effect of predation on moose numbers. Unrefined calculations suggest that annual production and loss in the moose herd are about equal. If the winter rate of kill for the large pack (one moose per 3 days) applies year around, this pack removes about 122 moose per year. The smaller packs (totaling five or six members) probably kill about a third as many, or 41, giving a total annual mortality of 163 moose. An estimated 564 are present in late May when calves are born. Since calves composed 25 percent of the summer observations, extrapolation suggests that at least 188 calves are produced, indicating that the population would remain stable or increase slightly. However, a precise evaluation obviously is not this simple.
A more thorough appraisal of moose-population dynamics requires consideration of two key figures, annual calf production, and percentage of yearlings in the total population. As used here, the term "short-yearling" is a calf in its first winter or spring, and "long-yearling" is an animal 1 to 1-1/2 years old.
Pregnancy rates are not known for the Isle Royale herd, so data from other studies must be used. In British Columbia, 75 percent of 80 adult uteri were pregnant, including some from before the end of the breeding season (Edwards and Ritcey, 1958). Pimlott (1959b) found that in Newfoundland 81 percent of 239 adults taken after November were gravid, and he believes this is less than the actual percentage. The assumed rate for Isle Royale moose is 80 percent, a conservative estimate, in view of the fact that heavily cropped populations usually are most productive.
Calf-production figures are derived from the pregnancy and twinning rates, and number of adult cows present in calving season. The estimated size of the herd on March 1 is 600 (page 98), including 102 yearlings (17 percent), but by calving season it should decrease to about 564 because of continued predation.
The known kill of the large pack is 19 adults and 17 calves in 110 days (based on data from three winters, table 11). If the small packs take a third as many moose, the kill for the entire population is 25 adults and 23 calves in 110 days. At this rate, the wolves remove 19 adults and 17 calves from March 1 to May 20, when calving season begins, so the herd then should contain about 479 adults and 85 yearlings. Half of the 479, or 239, would be adult cows, assuming an even sex ratio. If 80 percent (191) breed and bear an average of 1.19 calves each (see page 105), the calf crop is 227.
Yearling-total population ratios for Isle Royale are given in table 13, and the average annual ratio for early March is believed to be about 17 percent (page 106), which agrees with statistics from other areas. Figures from de Vos (1956) indicate that long-yearlings composed 10 percent of the population minus calves in the Chapleau Crown Game Preserve, and 13 percent in the general Chapleau District of Ontario. The ratio calculated from Knowlton (1960) for the Gravelly Mountains of Montana was 25 percent, for short-yearlings. Pimlott (1959b) found that reported ratios varied from 9 percent to 23 percent. Such variations might result from differences in methods of obtaining the figures. Some are based on summer observations, others on hunter-kill data and still others on winter aerial observations. Probably the study most comparable to the Isle Royale work is that of Spencer and Chatelain (1953). Pimlott calculated that short-yearlings composed 17 percent of their 9,436 winter aerial observations made in four Alaskan areas over a 3-year period. Most low ratios reported were from summer, whereas the Isle Royale figure applies in March, so it compares favorably with the others. This ratio is significant because it is an excellent indicator of annual increment to the herd. Mortality statistics demonstrate that very few individuals aged 1 to 5 are lost (table 18). As calculated above, an estimated 85 animals survive their first year.
Having estimated calf production and annual increment, we can compare them with expected annual mortality. Since the calculated kill is 25 adults and 23 calves per 110 days in winter (see above), annual adult mortality approximates 83 animals, assuming the same rate of adult kill year round. The rate of calf kill cannot be projected for the entire year because summer calves are so much smaller than calves in winter. If the rate is constant from November 1 to May 20, 42 calves are consumed in this period. An indication of summer calf loss can be obtained from the percentage of calves present in the autumn population. The only autumn sample taken showed that 22 percent of 150 moose were calves (page 104). Theoretically, 40 adults should have perished between May 20 and late October, when the survey was made, leaving 524. If calves composed 22 percent of the total population, then 148 calves survived; this indicates that approximately 79 died from May 20 to November 1. On this basis, annual mortality would be 83 adults and 121 calves (42 plus 79), or a total of 204. Since approximately 227 animals are believed to be produced each year, the herd would increase annually by about 23, on the basis of the above computations.
However, more substantial figures show that about 85 calves survive to their first year; 227 minus 85 equals 142 calves lost, 21 more than previously calculated. This discrepancy could result from a mistaken assumption that the winter rate of calf kill applies from November to May. Most likely more calves are taken in autumn and early winter, when they are smaller, more numerous and presumably more vulnerable. If this is true, a more realistic figure than 42 for calf mortality from November to May would be 142 minus 79 (summer kill), or 63.
The annual calf kill is a useful figure, but the statistics most indicative of the future trend in moose numbers are annual adult kill and annual increment. The calculated figures are 83 and 85 respectively. If these approximate actual numbers, the Isle Royale herd will remain stable for as long as they apply.
The annual-kill figure can be checked by comparing the approximate weights of animals killed with the total annual consumption (based on figures averaged from all three winter study periods). The large pack consumed a total of approximately 20,295 pounds in 110 days, or 184 pounds per day (page 77), and if the smaller packs ate a third as much, consumption for the entire population would be about 245 pounds per day, or 89,425 per year.
The weight of animals killed is more difficult to determine. Assuming that 85 adults are taken annually and that each provides about 800 pounds of food, then adults contribute 68,000 pounds per year. If 63 calves at 275 pounds are killed between November 1 and May 20, they provide 17,325 pounds. The estimated 79 calves taken between May 20 and November 1 should average about 81 pounds apiece—calculated from weekly calf weights given by Peterson (1955), Denniston (1956), and Dodds (1959)—so these furnish approximately 6,399 pounds. The three estimates total 91,724 pounds, which compares well with the calculated annual consumption. The close agreement is not important, since most of the figures are estimates; the significant point is that both numbers are within the same order of magnitude.
Another figure that compares favorably with production and loss statistics is the summer ratio of calves to total population (25 percent) based on field observations (page 103). The calculated calf production (227) is 33 percent of the estimated herd, but numbers undoubtedly dwindle rapidly during the first few weeks because of predation. Probably the loss rate declines as calves grow and provide more food. Since the summer calf ratio is an average of observations from about May 20 to September 20, it should be less than the percentage present on May 20 and more than the September ratio. Although the latter is unknown, the figure for November, based on 150 moose, is 22 percent. Thus the conservative estimate of the average summer ratio falls into line.
The following non-assessable factors could affect the production-loss calculations, but probably none is influential enough to destroy the worth of the proposed figures: the importance of beavers as summer food, possible waste of adult moose killed by small packs in summer moose mortality other than predation, and difference in wolves' summer food requirements. Regarding the latter factor, E. H. McCleery, who has maintained a wolf kennel for years, wrote me that he feeds his animals an estimated five-sixths as much in summer as in winter.
The computed proportion of calves in the summer kill does not correlate well with the wolf-scat analysis (table 10). Calf hair composed 48 percent of the occurrences from May to August, and adult hair constituted 16 percent. If 40 adults and 79 calves are killed between May 20 and November 1, calves furnish about 6,399 pounds of food, whereas adults provide about 32,000. There could be several reasons that the scat analysis might not accurately indicate absolute or even relative ratios of calves to adults consumed: (1) Calves are covered with a higher proportion of hair than are adults; (2) all hair is consumed from summer calf kills, whereas large chunks of hide are left at adult kills at least in winter; (3) a wolf could eat much meat from an adult without getting hair, but this would be difficult with a calf; and (4) when an adult is killed in summer, probably the wolves travel little until it is finished, so most scats would be left nearby; however, wolves probably finish a calf quickly and then continue, leaving a higher proportion of scats containing calf remains on trails. These and other possible biases indicate that scat-analysis figures are not a valid check on calculated kill rates.
Postulated seasonal trends in the moose herd are diagramed in figure 102. This model is not a precise estimate of actual numbers; rather it is an idealized scheme based on limited data. As such, it should be useful for considering the effects of wolves on moose numbers, even though future work may necessitate its modification.
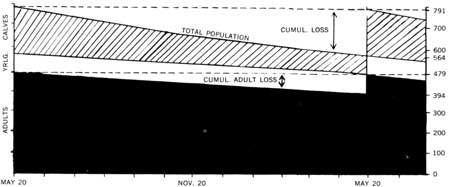
Figure 102—Seasonal trends in the moose herd.
The age-structure curve of the moose herd also can be plotted. Remains of 39 ageable adult moose were discovered at random and segregated into wear classes indicating relative age (table 18); these provide estimates of the percentage of mortality (from wolf predation and all other factors) occurring in each class. Assuming an annual increment of 85 yearlings and a mortality of 85 adults, one can determine the number of individuals in each wear class by subtracting the calculated mortality from the previous class, starting with 85 members in class I. When these are plotted on a graph, a profile of the age structure of the herd (just before calves are dropped) results (figure 103 and table 22). This total moose in each wear class, 493, compares favorably with the estimated population size just before calving season (564).
TABLE 22.—CALCULATED AGE COMPOSITION OF THE MOOSE HERD JUST BEFORE CALVING SEASON
| Wear classa | Number of moose remains found |
Percent of total mortalityb |
Mortality | Population |
| I | 1 | 2.56 | 2.17 | b85.00 |
| II | 2 | 5.12 | 4.34 | 82.83 |
| III | 1 | 2.56 | 2.17 | 78.49 |
| IV | 4 | 10.24 | 8.68 | 76.32 |
| V | 8 | 20.48 | 17.36 | 67.64 |
| VI | 8 | 20.48 | 17.36 | 50.28 |
| VII | 6 | 15.36 | 13.02 | 32.92 |
| VIII | 5 | 12.80 | 10.85 | 19.90 |
| IX | 4 | 10.24 | 8.68 | 9.05 |
| IXA | ... | ... | ... | .37 |
| Total | 39 | 99.84 | 84.83 | 492.80 |
bCalculated annual adult mortality and annual increment is 85.
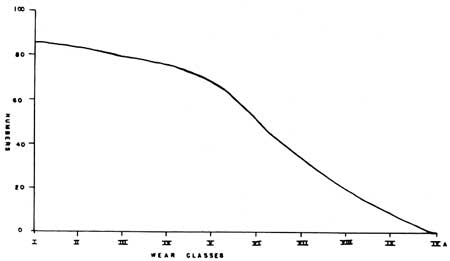
Figure 103—Profile of the age distribution of the herd.
The browse-moose-wolf complex can be summarized for the Isle Royale ecosystem in terms of weight, on the basis of data from this study and one figure from the literature. Since no attempt was made to measure browse consumption of moose, figures from other studies will be used. Hickie (1937) determined that a captive moose requires 25 pounds of browse per day, and Kellum (1941) found that captive animals that were supplied "unlimited" food consumed 40 to 50 pounds per day in winter and 50 to 60 in summer. He believes that summer consumption appears higher because of the high water content of summer foods. In addition, Palmer (1944) estimated, on the basis of tests with caribou, reindeer, and musk-oxen, that a 1,200-pound moose requires about 35 pounds per day. Because wild moose must gather their food, they probably do not consume as much as captive animals, so I will assume that an adult eats 25 pounds daily in winter and 35 pounds in summer.
The Isle Royale wolf population annually devours an estimated 89,425 pounds of moose (page 163), which equals about 112 adults at 800 pounds each. (Since browse consumption figures are based on adults, the wolves' consumption must be converted to adults only.) If the summer rate applies from May 1 to September 1 and the winter rate for the rest of the year, each moose eats about 10,325 pounds per year; the 12 would consume 1,156,400 pounds annually. Since the average Isle Royale wolf is assumed to weigh 72 pounds (page 77), the entire population should weigh about 1,512 pounds. The ratio of moose to browse is 7.7 percent; of wolves to moose, 1.7 percent; and of wolves to browse, .13 percent. Thus, yearly, about 762 pounds of browse are consumed for each 59 pounds of moose, in turn consumed for each 1 pound of wolf.
The above calculations demonstrate the tremendous energy loss that occurs from one trophic level to an other. However, since it takes an estimated 564 moose to produce the weight or number consumed, the annual weight of browse consumed is more realistically in the neighborhood of 5,823,300 pounds—or 3,851 pounds of browse per pound of wolf! The true amount of available or total browse, versus the amount consumed by the herd, is unknown.
Continued >>>
 Top
Top
Last Modified: Thurs, Jul 4 2002 10:00:00 pm PDT
http://www.cr.nps.gov/history/online_books/fauna7/fauna6.htm
![]()
